How to Solve Complex Fractions?

Maths as a subject is exceptionally fascinating. Almost every topic under the giant umbrella of ‘Mathematics’ has a practical, real-world application. One of the fascinating topics that Maths as a subject has to offer is ‘Numbers.’ It all started with some basics of numbers by the Egyptians, which was then expanded by the Greeks.

One of the major roles played in the evolution of concepts related to numbers, such as Natural numbers, Prime numbers, Complex numbers & Fractions, was by the Hindu-Arabic contingent, which eventually led to the discovery of the concept of the number ‘Zero’.

Moving on, as a result of human curiosity, it led to the discovery of more concepts like Whole numbers, Natural numbers, Rational Numbers, Irrational Numbers, and the list goes on…So let us jump right into the topic to learn numbers!

Origination of fractions

It was at the beginning of the 17th century that something very interesting happened. Humans discovered a means of ‘comparing whole numbers.’ They realized that we could break-up things into smaller parts & use them for our purpose.

For example, if we order a large pizza for dinner for a family of four, how can we divide the whole pizza into smaller parts so that each member gets at least two pieces to eat?

”Can we express the division of the pizza in smaller parts, numerically?”

”Is there an easier way of expressing one small piece of pizza, with respect to the whole pizza?”

As curious as the human mind is, we started finding out more ways to express ‘something, with respect to the whole.’ Fractions are a topic that can be best understood when ‘imagined’ or ‘visualized.’ It was then that we came up with the concept of fractions.

The word ‘fraction’ was originally derived from the Latin word ‘fractus,’ which means “broken.” If we try to understand the concept in very simple terms, it tells about ‘how many parts of a whole do we have.’

Coming back to the example of a pizza, if the member ‘A’ of the family has 2 parts of a pizza, which was initially divided into 8 different parts, we can say that member ‘A’ has 2 out of 8 parts of the pizza.

The easiest way of expressing this is 2/8. If we simplify or reduce this expression, then it comes down to 1/4. The position of ‘1’ is called the ‘numerator’ & the position of ‘4’ is called the denominator. Due to its ease of understanding, this method was accepted and widely adopted all across the world.

Different types of fractions.

1. With time & research, there was an inclusion of a few more types of fractions. As we have already seen above, a fraction consists of a numerator & a denominator, so now let us dive deeper into the different types of fractions. In total, there are 6 types of fractions, but out of them, the first 3 define the type of ‘single fractions’ while the other 3 determine the comparison between multiple fractions(Two or more).
2. Fractions are segregated into 3 main types as follows.

• Proper Fractions.
• Improper Fractions.
• Mixed Fractions.

Proper Fraction

It is defined as a type of fraction where the numerator is less than the denominator.

i.e., Numerator < Denominator. For a proper fraction, the value of the fraction after simplification is always less than 1.

For example: 1/4= 0.25.

Improper Fraction

It is defined as a type of fraction where the denominator is less or equal to the numerator.

i.e. Denominator <= Numerator. For a proper fraction, the value of the fraction after simplification is always greater than or equal to 1.

For example: 10/5= 2,1 etc.

Mixed Fraction

It is defined as the type of fraction, which is a combination of a natural number and fraction. It is more commonly known as an improper fraction.

For example 2 (⅚).

A mixed fraction is a type that generally scares the students at first, but it can be easily simplified as follows: Natural Number(2) x Denominator(6) + Numerator(5).

• Comparing two or more fractions.

Now that we have discussed the three main types of fractions let us discuss a few more types where we will get to know how the comparison of two fractions occurs. The next three types of fractions are as follows.

1. Like Fractions.
2. Unlike Fractions.
3. Equivalent Fractions.

1.Like Fractions.

• The type of fractions that have the same denominators are called ‘like fractions.’

For example, 4/2, 7/2, 8/2, 9/2 are like fractions.

• These fractions can be easily simplified, as all the denominators here are the same. Suppose we need to add all the above like fractions, then.

3/2 + 5/2 + 7/2 + 9/2 = (3+5+7+9)/2 = 24/2 = 12.

1. Unlike Fractions:

• The type of fractions that have unequal denominators or the ones that have different denominators are called unlike fractions.
• For example, 1/2, 1/3, 1/4, 1/5 are unlike fractions.
• These fractions are generally a bit lengthy to simplify; hence we first need to factorise the denominator, and then we simplify them (if it’s a case of addition and subtraction).

Let us suppose that we have to add 1/2 and 1/3. After this, we will have to find the LCM of 2 and 3, equal to 6.

Now we need to multiply 1/2 by 3 and 1/3 by 2, both in numerator and denominator.

The fractions become 3/6 and 2/6.

Now, if we add 3/6 and 2/6, we get.

3/6+2/6 = 5/6.

1. Equivalent Fractions: It is a type of fraction where two or more fractions have the same result after they are simplified for which they represent the same portion of the whole.

For example, 1/2 and 4/8 are equivalent & 1/3, and 9/27 are equivalent.

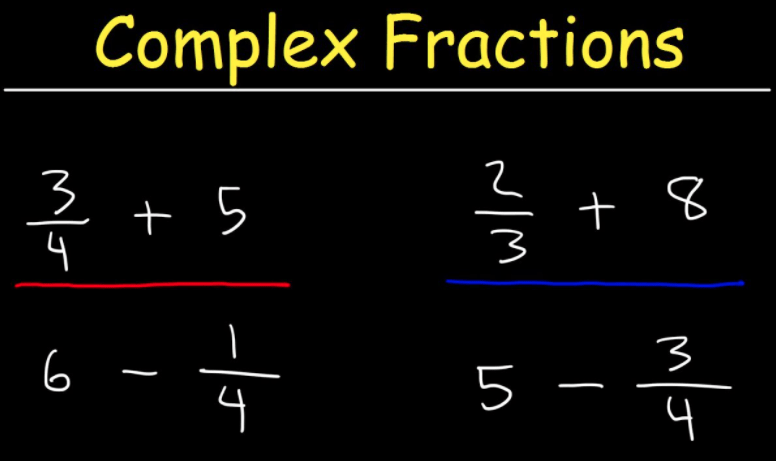
• Complex Fractions.

It is perhaps one of the most interesting topics, but slightly intimidating if we look at it from a student’s perspective. Earlier, we discussed the different types of fractions & their comparisons. Now, we will discuss some operations that we can perform on them.

In simple words, a complex fraction can be defined as a normal fraction, which consists of one or more fractions, both in the numerator & denominator. Since the complex fractions are stacked over each other, they are also called Stacked Fractions.

For example:

32/(25/2).

Here, we can clearly see that there is a fraction in the numerator and the denominator. Some very simple steps can be used to solve & simplify the above fraction.

Here, we apply the division rule by multiplying the numerator by the reciprocal of the denominator.

herefore, 32x 2/25= 64/25.

It was a very simple example where we have used only basic fractions to simplify them down to a single reduced fraction.

Let us look at a slightly different example, where we are adding another operation to the fraction.

(1+1/x)  /(1-1/x).

In this particular example, we will have to reduce the terms in both the numerator and the denominator, resulting in a single fraction.

Here are the steps.

1. Here we can see that both in the numerator and the denominator, we can see that there is an ‘x.’ Hence, we consider the Least Common Denominator(LCD), which in this case is, ‘x.’
2. Now, we multiply both the numerator & the denominator with the LCD.

{x(1+1/x)} /{x(1-1/x)}= (x+1)/(x-1).

Therefore, we get our final simplified fraction as.

(x+1)/(x-1).

• In both the above examples shown, we solved them using two different methods.
• The first method required applying the division rule, whereas, in the second method, we use the LCD(Least Common Denominator) to reduce it down to a single simplified fraction.
• Both the examples can be solved using both the methods; however, we can see that the 2nd method is comparatively less lengthy & is easier to solve!
• As the number of operations to be performed in the fraction increases, it is generally easier to solve using the LCD method.

Topics like complex fractions are more about understanding the rules used & accordingly following the steps to reduce them down to the simplest fraction possible. But to have a deep understanding of fractions, it is crucial to be able to relate it to real-life examples.

We have designed our modules in accordance with an understanding of child psychology amalgamated with technology, making it almost effortless to understand fractions!